Keyboard Shortcuts?f

×
  • →Next step
  • ←Previous step
  • ↓Skip this slide
  • ↑Previous slide
  • mShow slide thumbnails
  • nShow notes
  • hShow handout latex source
  • NShow talk notes latex source

Click here and press the right key for the next slide.

(This may not work on mobile or ipad. You can try using chrome or firefox, but even that may fail. Sorry.)

also ...

Press the left key to go backwards (or swipe right)

Press n to toggle whether notes are shown (or add '?notes' to the url before the #)

Press m or double tap to slide thumbnails (menu)

Press ? at any time to show the keyboard shortcuts

 

Expected Utility

in this lecture we're going to explore how some formal methods borrowed from decision theory might enhance our understanding of the discoveries about action
we're also going to explore, conversely, how those discoveries might bear on our use of these formal models.
Although this is going to seem quite dry at the beginning, when i taught this course in the past this was one of the areas that most interested people. So do bear with me.

decision theory

How do rational agents decide which of several available actions to perform?

simple question: How do rational agents decide which of several available actions to perform ?
One is a lottery ticket, the other a coin toss
According to Lanchester’s novel Mr Phillips, I’d need to buy a lottery ticket at most 11 minutes before the draw in order for my chance of winning to be greater than my chance of dying before the draw.
Most people here (16-24) could buy a ticket as much as 68 minutes before the draw to achieve the same result.
Expected utilities can be derived from desirabilities and probabilities. One procedure for choosing an action is to compute expected utilities (in the way illustrated) and then perform the action with the highest expected utility.

I don’t understand expected utility

Is there anything you do not understand? Have a chat with the person next to you.
I can illustrate it using gambling
First illustration is a simple bet.
Which gamble would you prefer? If you are like me, you are interested in the expected gain ...
Second illustration is about which of two bets you should select if you can only choose one.
OK so that was an illustration with money.
I used money to simplify the idea of expected utility.
But what we are really interested in is preferences.
this is gambling, and gambling is easy to understand.
But the thing is that if you understand gambling, you also understand decision theory. Because decision theory is just like the value of a bet with one tiny difference.
When we're thinking about gambling, we're trying to work out objectively what the probabilities are, and we're trying to work out objectively what the market value of the outcomes are. But me, the market value is probably different from my own subjective value.

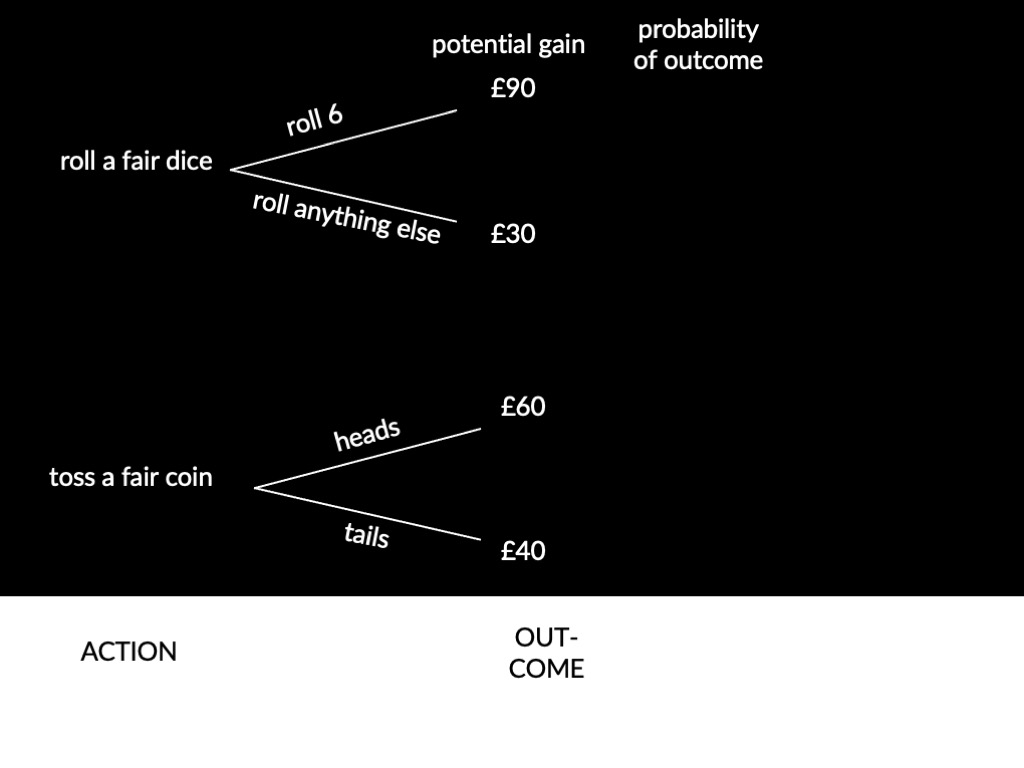
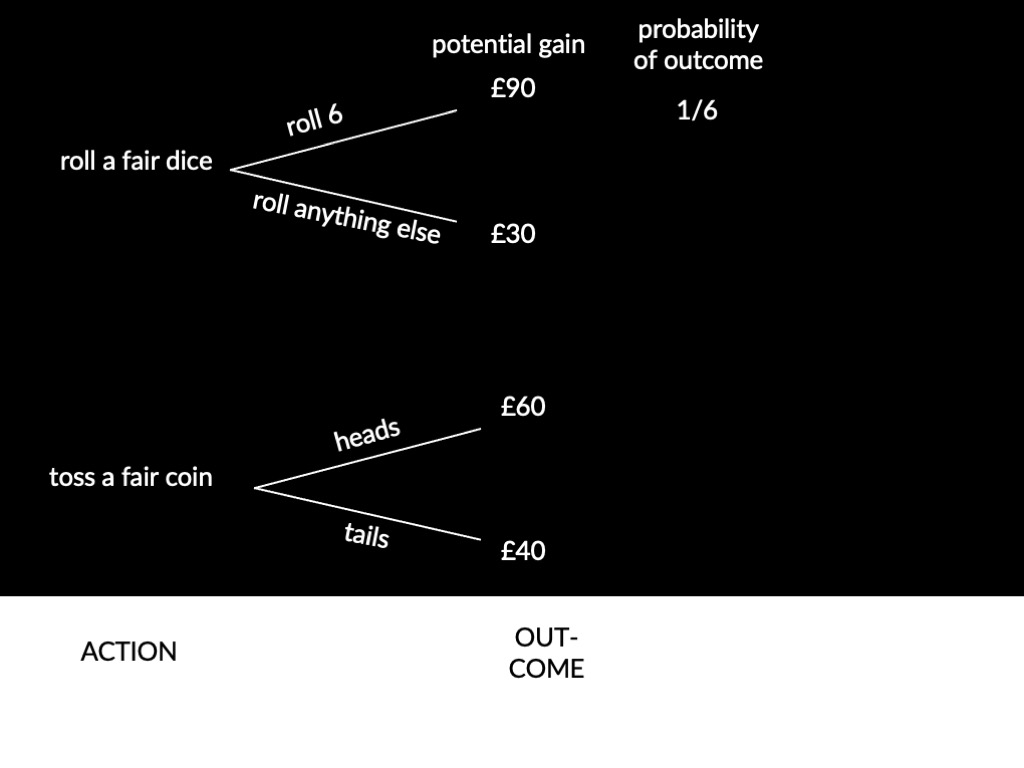
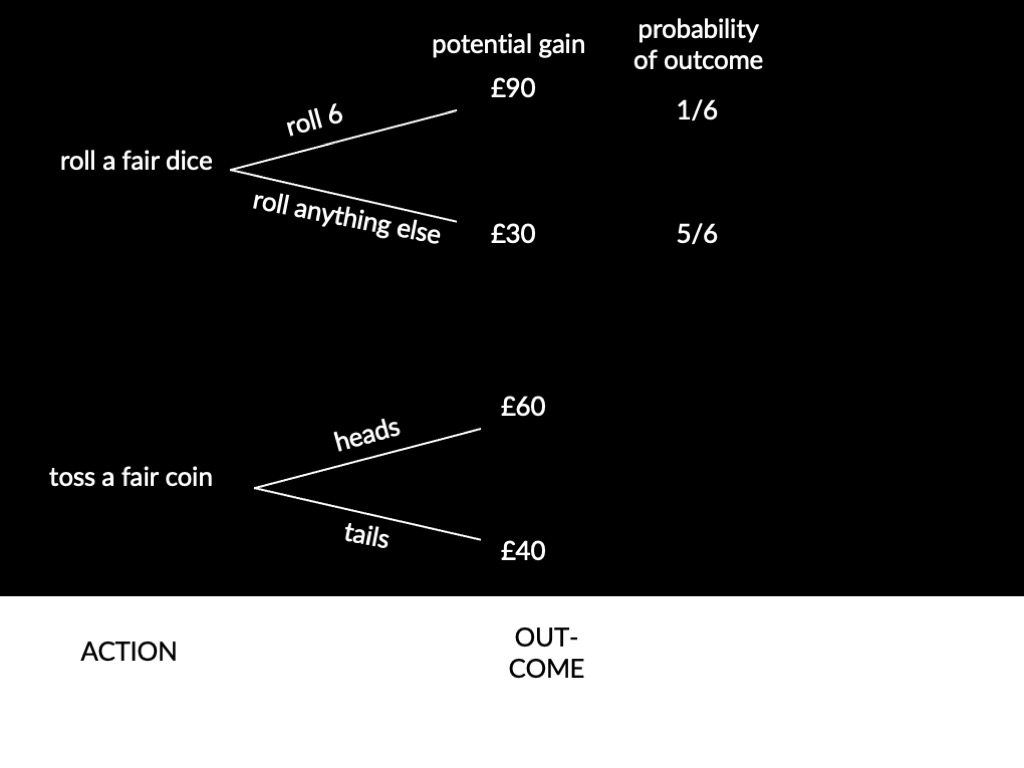
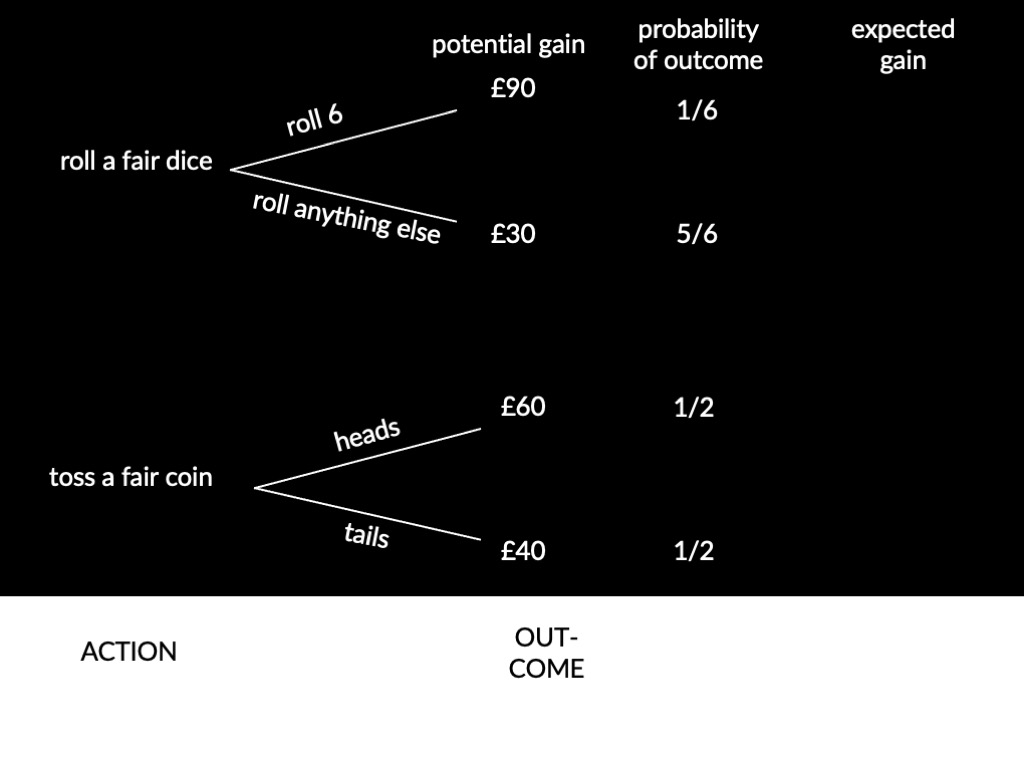
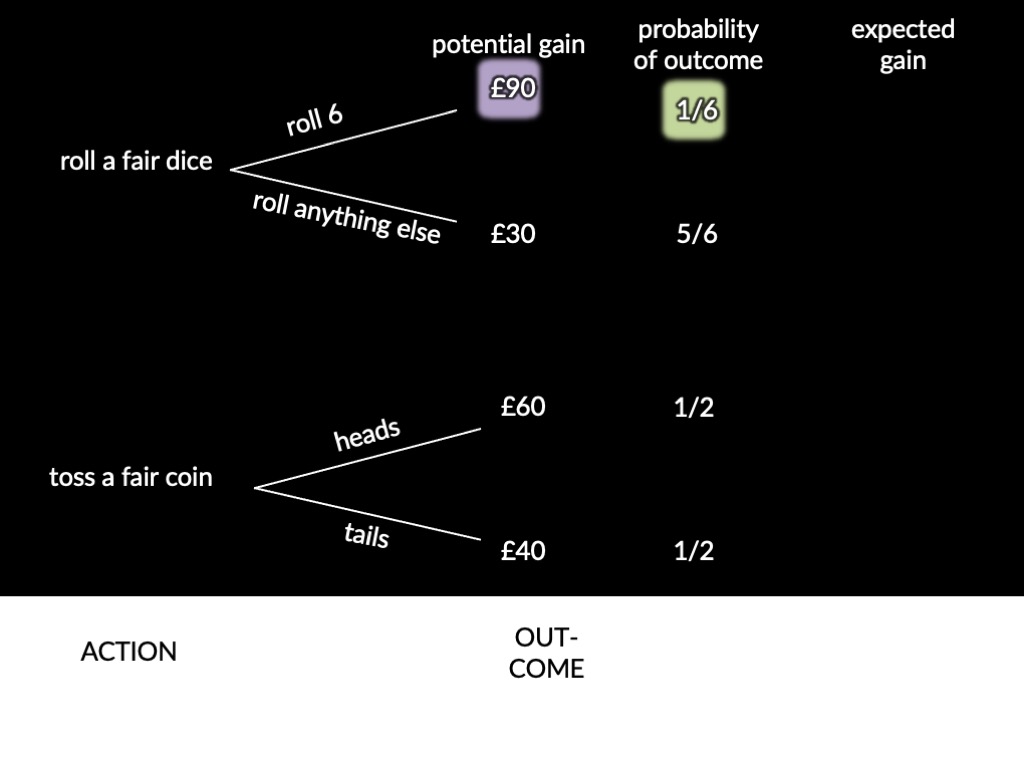
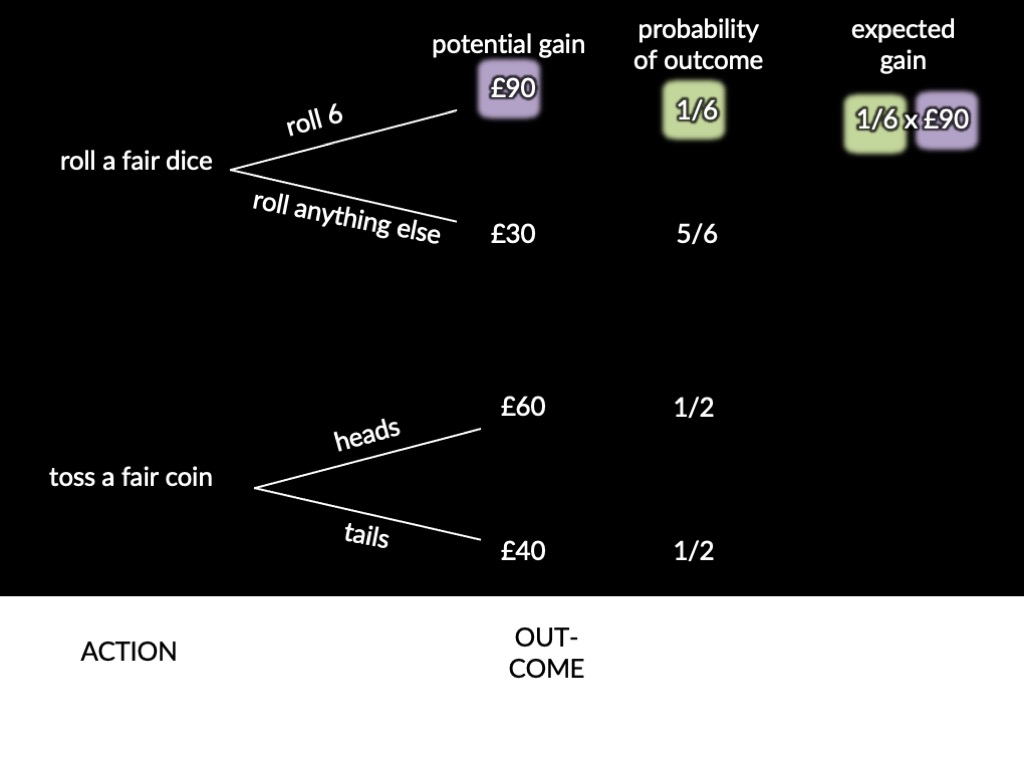
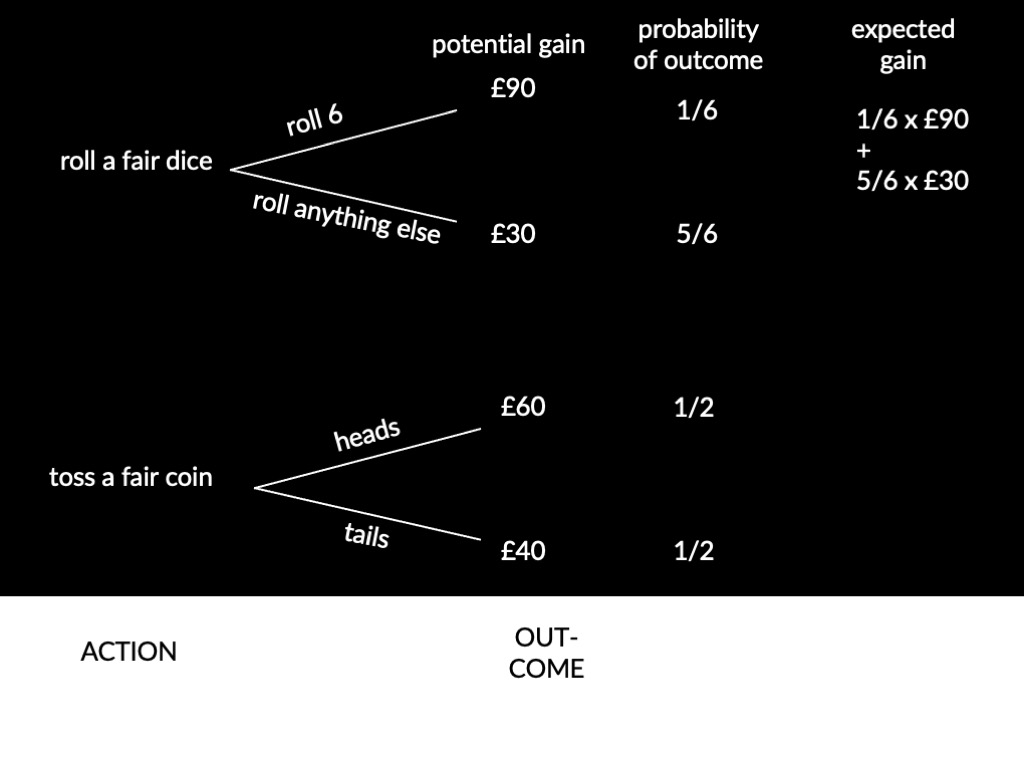
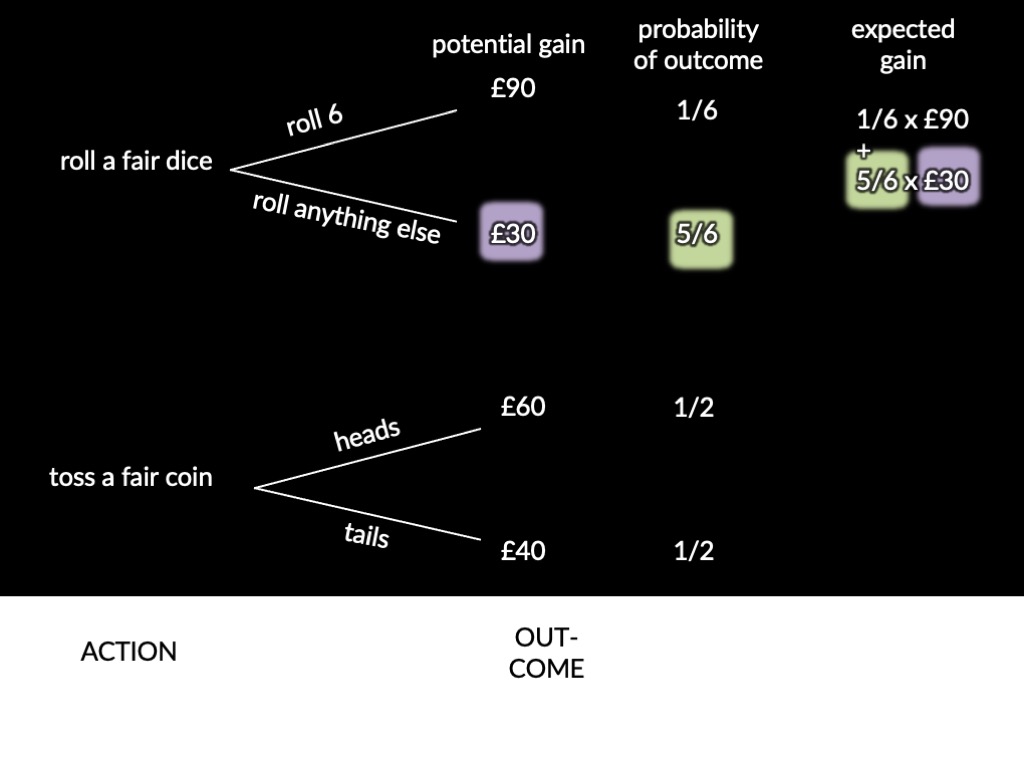
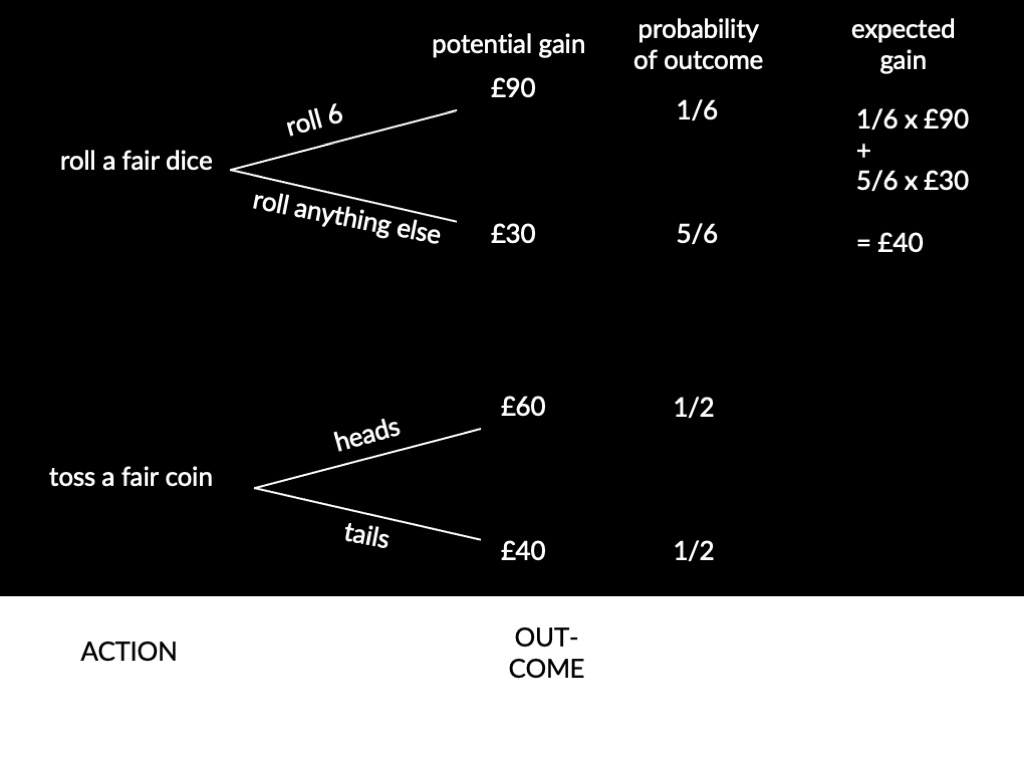
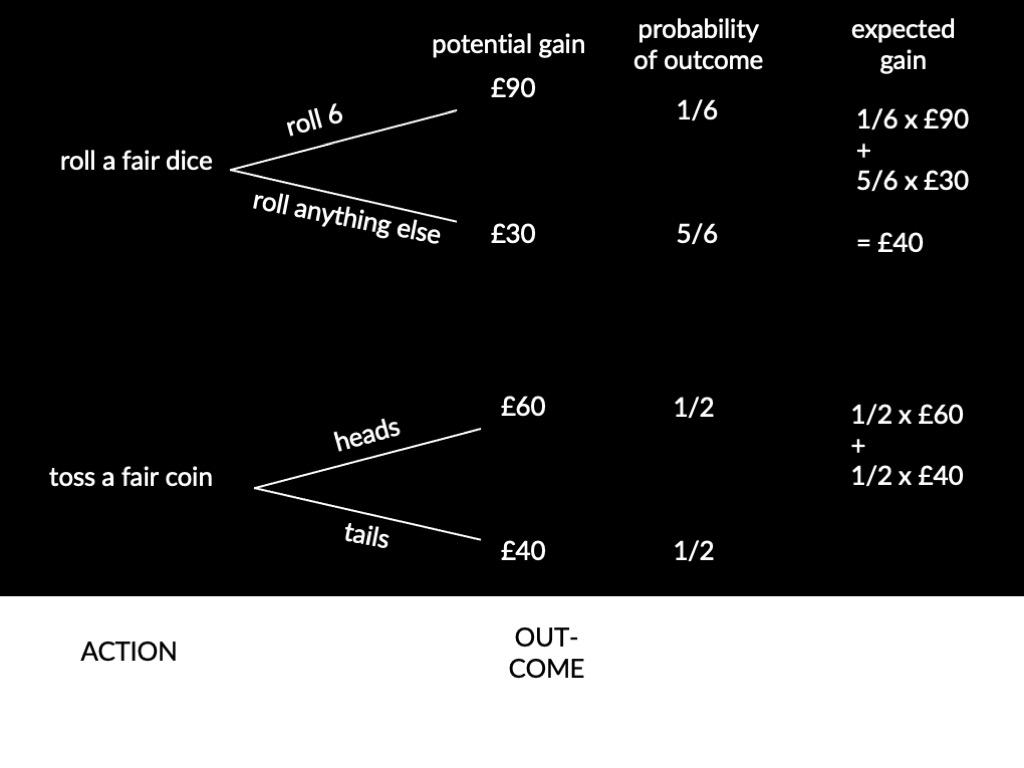
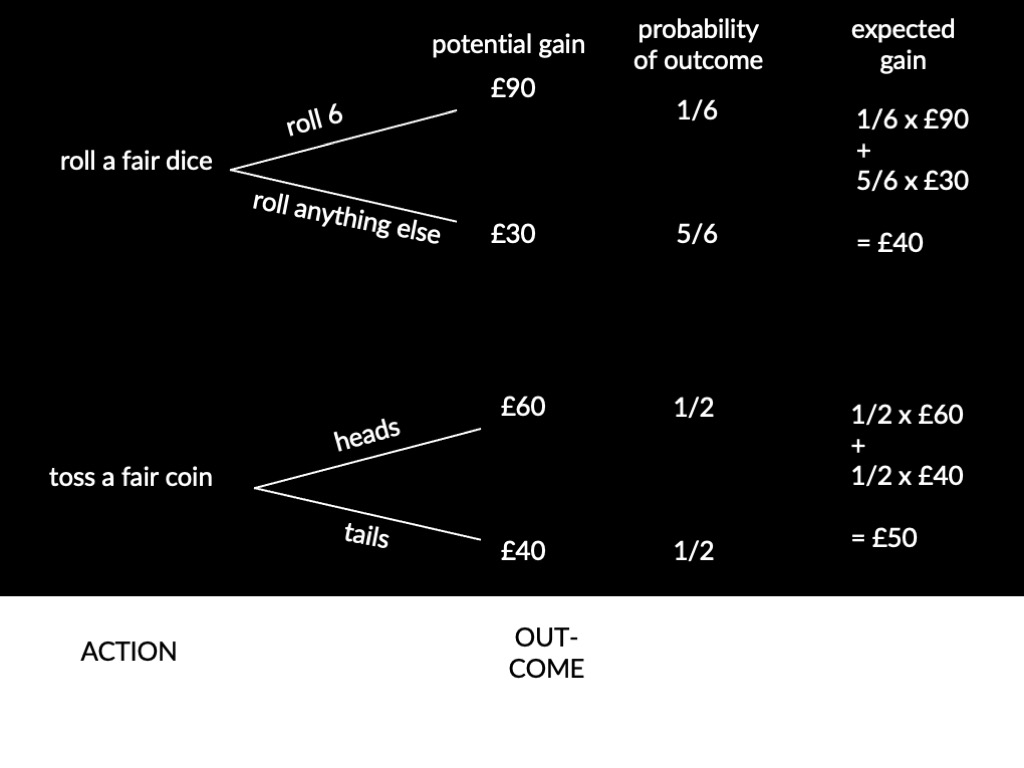
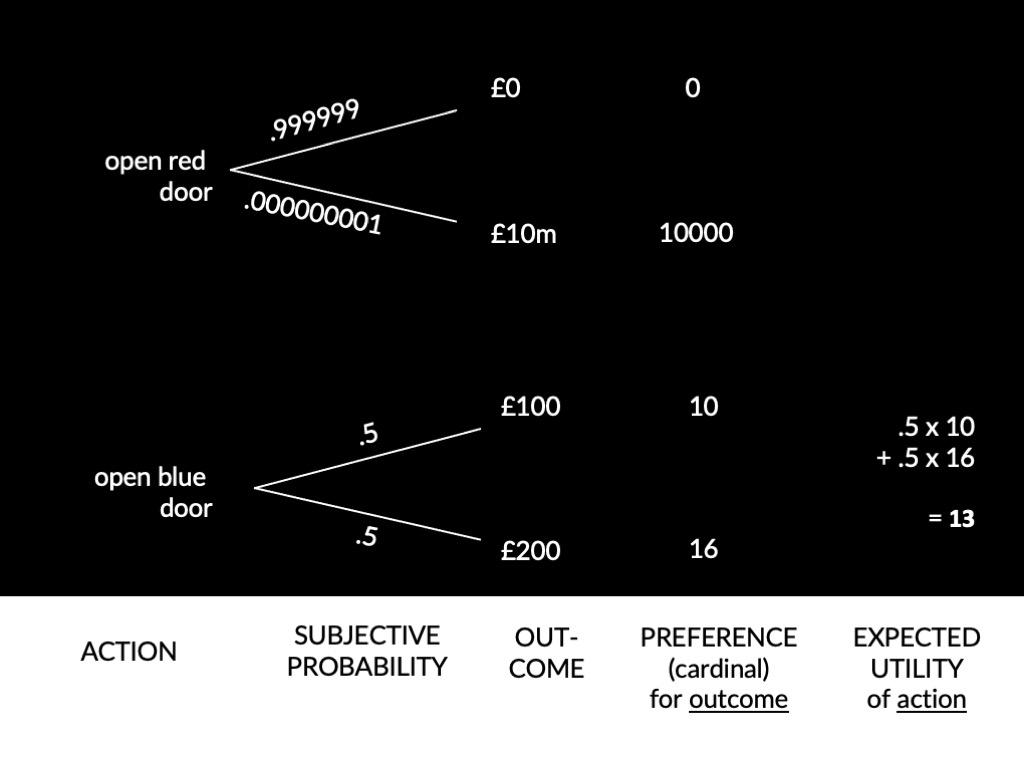
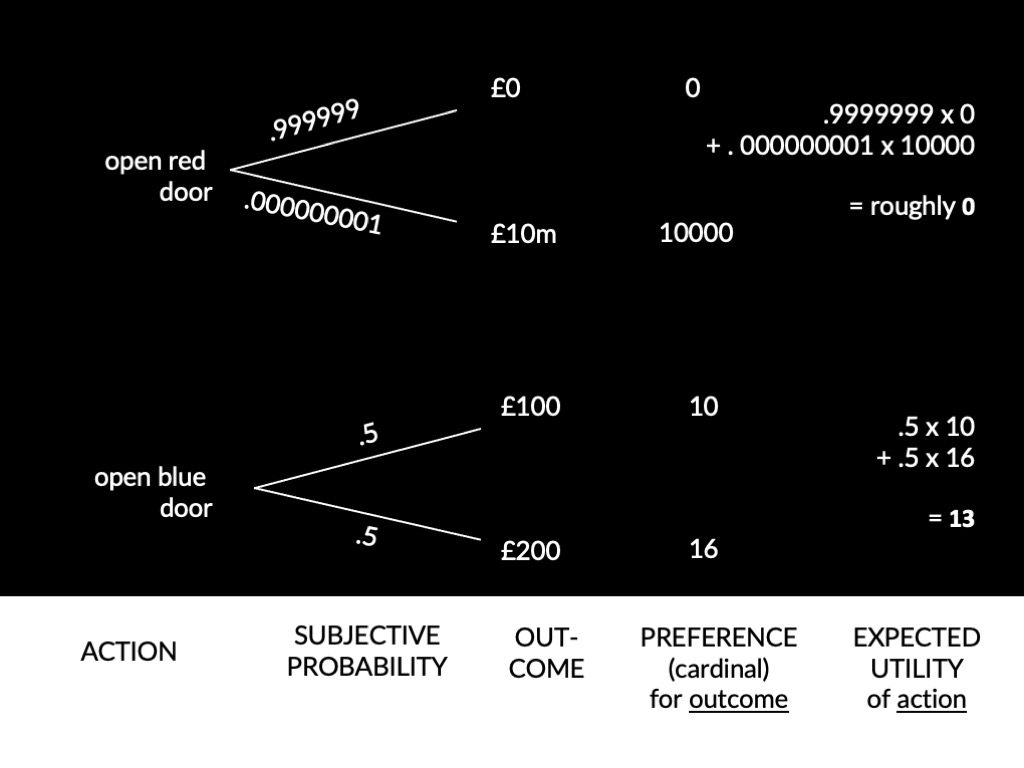
expected utility
is a bit like
the value of a bet

This is because your preferences do not necessarily vary linearly with monetary amounts. Once you have the first £100, the next £100 might not make nearly as much difference to you and so it might be rational to care a lot less about whether you have it.

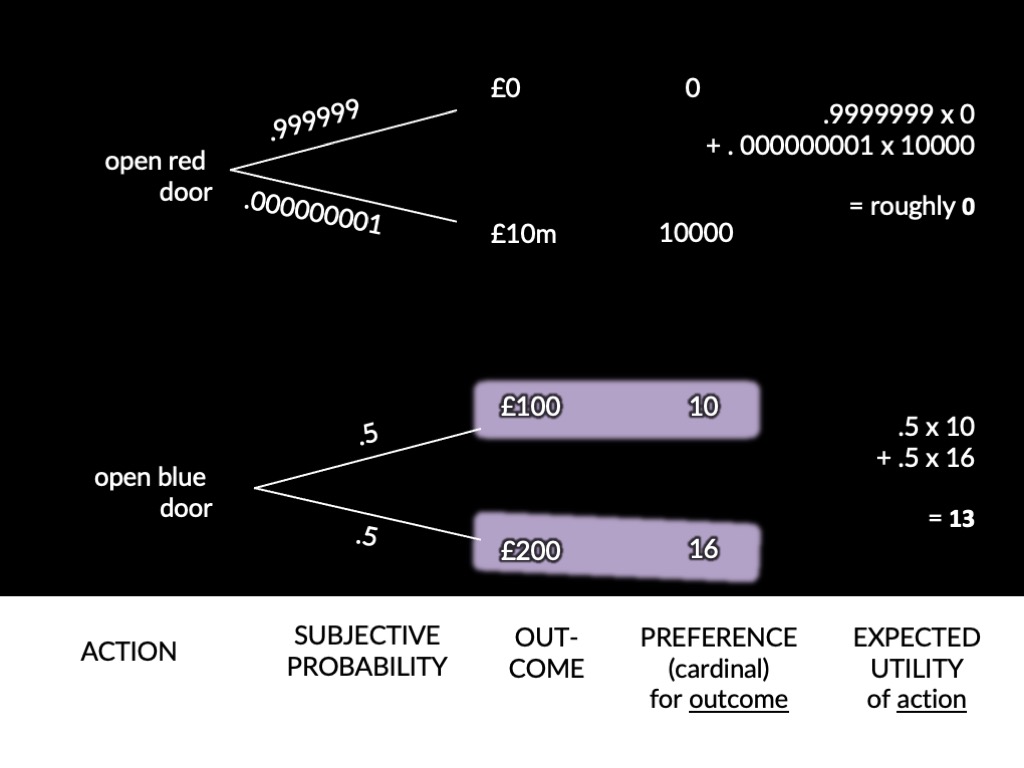
Terminology

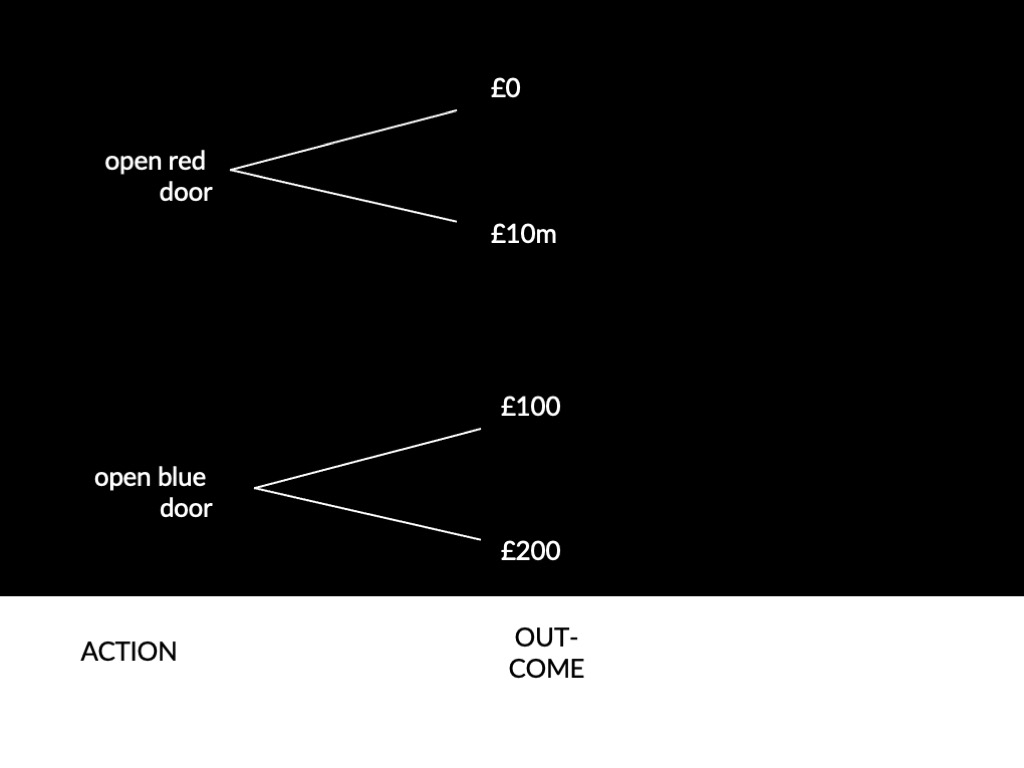
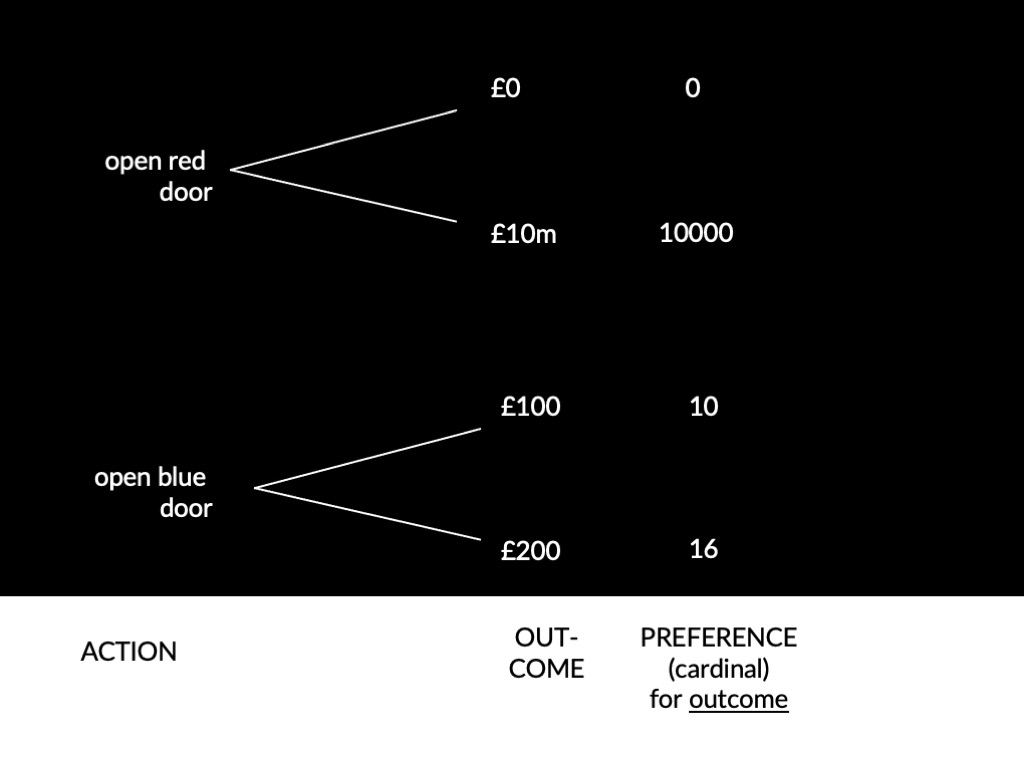
actions have outcomes

(Jeffrey: outcome = ‘consequence’)

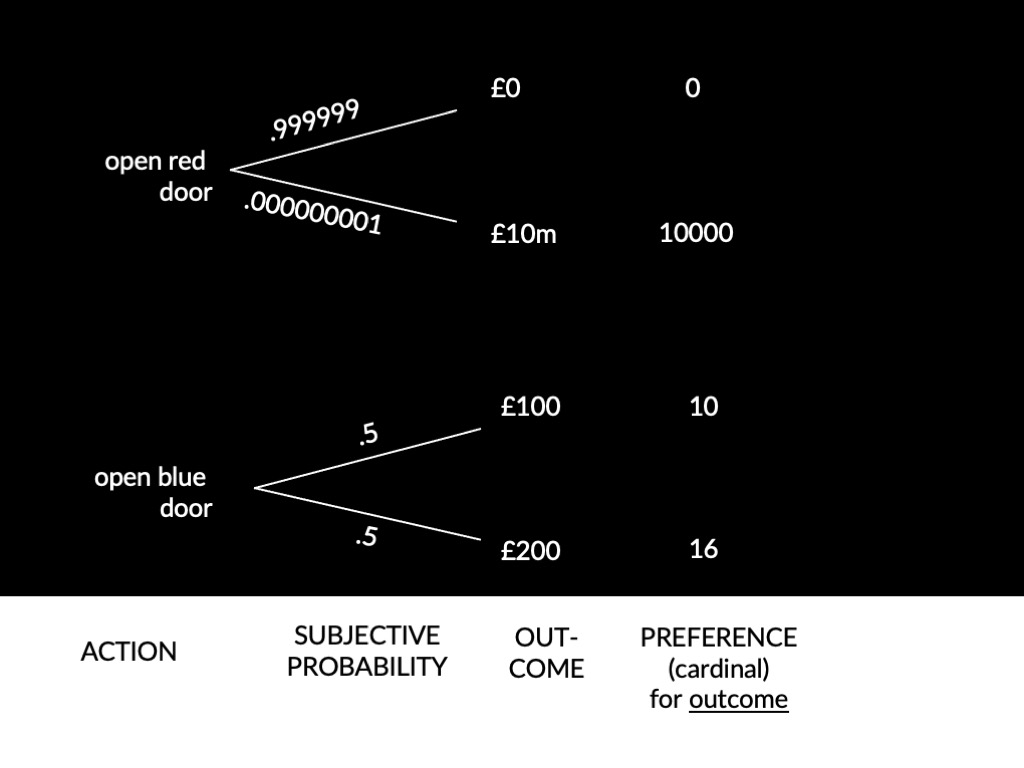
which outcome an action causes depends on **conditions**

subjective probabilities attach to conditions.

preferences rank outcomes

expected utilities attach to actions.

(Jeffrey: expected utility = ‘estimated desirabilities’)

Jeffrey (1983)

Make sure you understand the terminology and can relate it to the scenario.
Be sure to use the terminology consistently, and with precision, in your writing.
It is important to understand that we haven’t done anything more than introduce a way of representing things.

so far: the representation

next step: the theory

We have not yet considered a theory. But understanding the representation is an essential first step towards von N & Mo’s objective.

‘we wish to find the mathematically complete principles which define “rational behavior” for the participants in a social economy, and to derive from them the general characteristics of that behavior’

(Neumann et al., 1953, p. 31)

Why is what we have done so far not sufficient?
(Because we have not anchored preference or subjective probability to subjects.)
(We have not said anything at all about what it is for someone to have a particular preference, nor what it is for them to assign a particular subjective probability to a given condition.)
So what we've done so far is just talked about representation. We just talked about purely formal representation. We haven't done what von Neumann and Morgenstern do, which is to try and say these are mathematically complete principles which define rational behaviour. The reason we haven't done that, I think, is relatively simple. We haven't connected up the decision theory to the person.
So what we've got is a purely formal way of representing things. We haven't got any way of connecting that to any particular person. And therefore we can't say that we have found mathematically complete principles which define rational behaviour.
But just here I want to stop for 90s.
There's two things that you might discuss with the person next to you or behind you. It might be, why is it that we've only got the representation? What's the thing that we need?
I've told you what we need is the connection between the representation and the person. But it might be that that doesn't make a lot of sense at this stage.
On the other hand, if that's completely clear to you, then the question is how do you take the next step? What's the next step that I'm going to offer you in connecting up the formal representation to the individual person and their actions?
90s go.